What is a Present Value?

Present value is like a time machine for money…

By understanding present value, you see what your future money is worth today. On the flip side, it also helps you know what the money you have today could be worth in the future. Your time machine can go to the past and the future!

The present value is vital for a few different reasons. First, it helps you understand how much a future amount of money is worth in today’s dollars, based on a chosen discount rate. It is essential when you are calculating how much you may need to invest today to reach a target amount in the future given an expected rate of return –– financial advisors often run these types of calculations for retirement planning.

You can also use the PV to calculate the future value of your cash flows. If you know what you have today, and you have a reasonable understanding of the expected rate of return, then you can calculate the future value of your money. For example, if you have $10,000 that you invest for five years at a 5% annual rate of return, you can calculate the expected future value –– $12,762.82. (Future returns are never guaranteed; all investments carry risk.)

Also, companies and investors often use present value as the basis for calculating net present value, which is an estimate of the current value of all future cash flows (both in and out). It gives them a way to evaluate and compare different investment opportunities.

Present value and future value are different sides of the same coin, and you need each one to calculate the other –– in addition to the discount rate.

Present value tells us that $1 today is worth more than $1 tomorrow. Why? In general, inflation will chip away at the value of your money over time. It means that whatever money you have today will decrease by the amount of inflation annually so that in the future, it will be worth less than today.

You also need to take into account the time value of money. That’s another way of saying that money today is worth more than the same amount of money in the future because of the potential to earn a return on it. For example, let’s say you have $5,000 today and invest it for three years at a 5% rate of return. In three years you’ll have $5,788. That’s how the time value of money makes $5,000 today worth more than $5,000 in the future.

Future value is the value of an asset in the future based on a specified rate of return (discount rate). Again, this is simply the flipside of PV. For example, let’s say you have $500 today (present value) and invest it for five years (number of periods) at 6% interest (discount rate). It gives you a future value of $669.

There are four essential elements in the equation: present value, future value, number of periods, and the discount rate. You always need the discount rate and the number of periods and then use PV to find FV or vice versa.

The formula for PV looks like this:

PV = FV/(1+r)n

The explanation for each element is:

PV = the present value in today’s money FV = the projected future value of the money r = the expected rate of return, interest rate, or inflation rate. Also known as the discount rate n = number of periods

As an example, let’s say that in 10 years, you want to have $20,000 available for your 10th anniversary trip to Tahiti. It is the future cash flow you want to have. You assume that you can get a rate of return of 5%. How much money do you need to put in the investment today (PV) to hit that future value of $20,000?

In this example: PV = $20,000/(1+5%)10 or $12,278.26.

Calculating PV in Excel

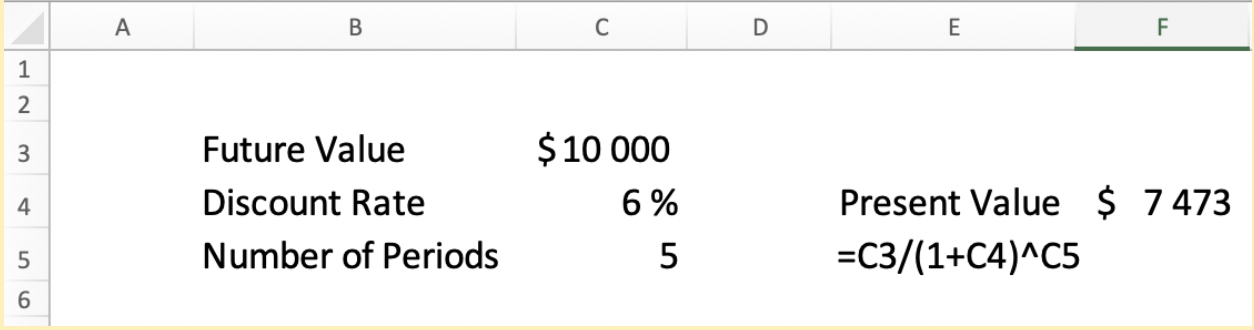
You can easily calculate PV using excel, and there are two different ways to do it. The first is useful when you are solving for single cash flow (a single payment instead of multiple payments over time). For example, let’s say you want to save for a car for your child. In 5 years, you know you’ll want to have $10,000 saved up (future value), and you believe you can get a 6% rate of return. You need to know how much you should put in your account today (PV) as a one-time payment and have it grow to $10,000 in 5 years.

For single cash flows, you can manually enter a formula based on the variables given. Using the same numbers from the example above, you end up with a formula of =C3/(1+C4)^C5.

Excel also has a built-in PV formula. It is useful when you want to know the present value for multiple cash flows. An example of this would be an Annuity –– a financial product that is to fund fixed payments during retirement. To use this functionality, you need to set your data up differently.

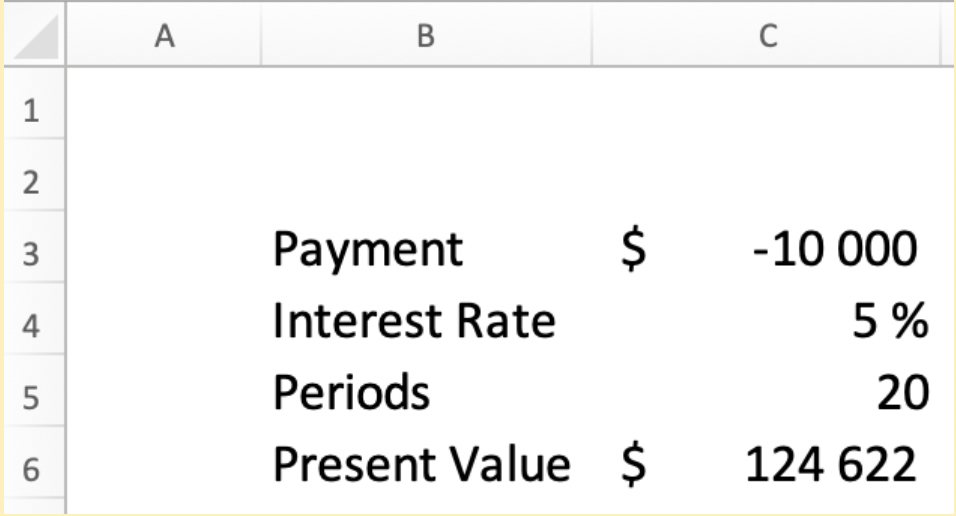
Let’s assume you have an Annuity that pays you $10,000 per year (multiple cash flows) for the next 20 years at an interest rate (discount rate) of 5%.

The function looks like this in excel:

Below are the explanations for the variables in the formula:

rate = the interest rate. In this case, it is 5%.

nper = the number of periods. Here it is 20 years.

pmt = this is the payment or outgoing cash flows. Here it is $10,000. One thing to note is that you should enter your payment as negative since it is outgoing cash flow. It gives you a positive present value. If you don’t, then you’ll end up with a negative PV.

fv = you leave future value as 0

type = there are two choices here. 0 is for the end of the period, and one is for the beginning of the period. It is when the payment is made. In this example, we are using the end of the period since this is a regular annuity.

You can see that even though the accumulated value over 20 years of all the payments is $200,000, the present value is only $124,622.

Present Value (PV) is the value in today’s dollars of a future amount of money. It’s a useful tool to help you understand how much you may need to invest for retirement or for any future purchases where you know an approximate target future value and an expected rate of return.

The concept builds on the fact that money today is generally worth more than the same amount in the future. Remember your grandparents talking about how going to the movies and getting popcorn cost them less than $1! In many cases, inflation eats away at purchasing power over time. So $100 today will not buy you the same amount of groceries as $100 in 10 years.

The present value calculation shows you the value of that future money in today’s dollars and helps you plan accordingly. You typically have two things to consider when using present value to help you make decisions. One is inflation, and the other is the time value of money.

As an example, let’s say you are given the choice of $10,000 today or $10,000 in seven years. First of all, we understand that money won’t be worth as much in seven years, simply due to inflation. Let’s assume inflation is at 2%. After adjusting for inflation, the future $10,000 is only worth about $8,706 in today’s money. It means the future $10,000 loses almost 13% of its value in today’s money.

But there’s another angle to consider. What else could you do with that $10,000 over the next seven years? You could invest the money. Let’s assume over the same seven-year period; you believe you can get a 7% annual return. After seven years, your original $10,000 would be worth nearly $16,057! (Investment returns are never guaranteed; all investments carry risk) So not only is your money worth less in the future due to inflation, but you could also miss out on increasing your money by investing it. Using present value allows you to see how much more your money is worth to you today than in the future.